S.A.T. Inequalities

This one comes right out of the S.A.T.s.

Problem:

Each of the following inequalities is true for some values of x EXCEPT

Solution:

The process of elimination is perfect for this problem. A quick look eliminates (A) as a possibility. Anyone could think of a value for x that would make (A) true; for instance, if x = 2, then x² = 4 and x³ = 8, so we can cross of choice (A).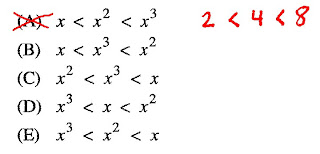
What other kinds of numbers are there? What numbers are "tricky"? Fractions and negatives. If x = 1/2, then x² = 1/4 and x³ = 1/8. That fits with the last inequality, so we can cross off choice (E).
Letting x = -2 makes x² = 4 and x³ = - 8. Ordering those gives us x³ < x < x², so we can cross off choice (D).
Now we only have two possibilities left, and they both look kind of goofy. If you were running out of time on the S.A.T.s, you could just guess between the two earning an expected value of 3/8 of a point, but we're not running out of time. We've tried positive integers, negative integers and fractions, so what's left: negative fractions. If x = -1/2 then x² = 1/4 and x³ = -1/8. That lines up as x < x³ < x², just like choice (B).
Therefore, the only inequality that will not be true for a value of x is choice (C).